This calculator simplifies terms that may contain fractions, brackets or powers.
Terms">
What is a term?
Term is a word for quite all stuff consisting of numbers, variables and arithmetic signs. This means that
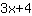
as well as

as well as

are terms.
A term containing a square root sign is called root term. A term containing a fraction is called fractional term.
A special set of terms are the polynomials consisting only of numbers and powers of x.
A term is a calculation consisting of numbers and variables . Variables are letters standing for numbers. Normally it is not known for what numbers the variables stand. Therefore one cannot simplify terms arbitrary far.
What are terms needed for?
To be able to do calculations with things you don't know. For example, you may sell wafers for
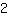
dollars and cake for
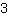
dollars. How much money do you earn? Well, this depends on how much you sell. For w sold wafers, you earn
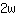
dollars. For k sold cakes, you earn

dollars. So you earn
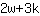
dollars, and here you got a term. If you now sell e.g.

wavers and
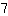
pieces of cake, you simply hafe to insert

for w and
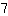
for k and find that you earned
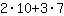
dollars, i.e.

dollars.
How to simplify terms?
You may e.g. put all appearing numbers together.
Example:
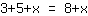
You can also put stuff together belonging to the same variable, e.g.
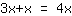
or
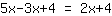
.
If different variables appear, simplifying is not always possible. E.g.,
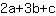
is not possibly simplified without knowing something about a, b and c.
What is expanding?
If a term is in brackets and this number gets multiplied by something, you can multiply every summand of the term (this means: The parts to be multiplied are separated by + or - signs. Example:


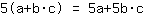
How can I understand what is not allowed with terms?
Simple rule: Whatever is right for every number is also right for terms.
Can I see more examples?
Of course. This is Mathepower. Just enter your exercise and Mathepower will simplify your term.
What does this program do?
It simplifies terms step-by-step. Mathepower has calculating scripts for nearly every part of school mathematics that help you to solve your exercises or verify solutions.